Cylindrical system

Système cylindrique (Fr) Sistema cylindrico (It).

Definition

The cylindrical system contains non-crystallographic point groups with one axis of revolution (or isotropy axis). There are five groups in the spherical system:

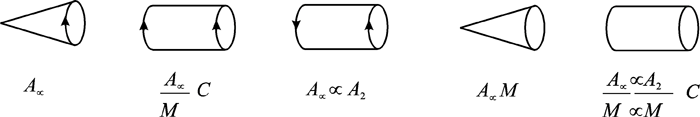
Hermann-Mauguin symbol	Short Hermann-Mauguin symbol	Schönfliess symbol	order of the group	general form
[math] A_\infty[/math]	[math]\infty[/math]	[math]C_\infty [/math]	[math] \infty[/math]	rotating cone
[math] {A_\infty \over M}C[/math]	[math] {\bar \infty}[/math]	[math]C_{\infty h} \equiv S_{\infty} \equiv C_{\infty i}[/math]	[math] \infty[/math]	rotating finite cylinder
[math] A_\infty \infty A_2[/math]	[math] \infty 2[/math]	[math]D_{\infty }[/math]	[math] \infty[/math]	finite cylinder submitted to equal and opposite torques
[math] A_\infty M[/math]	[math]\infty m[/math]	[math]C_{\infty v}[/math]	[math] \infty[/math]	stationary cone
[math] {A_\infty \over M} {\infty A_2 \over \infty M} C[/math]	[math] {\bar \infty}m \equiv {\bar \infty} {2\over m}[/math]	[math]D_{\infty h} \equiv D_{\infty d}[/math]	[math] \infty[/math]	stationary finite cylinder

Note that [math] A_\infty M[/math] represents the symmetry of a force, or of an electric field and that [math] {A_\infty \over M}C[/math] represents the symmetry of a magnetic field (Curie 1894), while [math] {A_\infty \over M} {\infty A_2 \over \infty M} C[/math] represents the symmetry of a uniaxial compression.

History

The groups containing isotropy axes were introduced by P. Curie (1859-1906) in order to describe the symmetry of physical systems (Curie P. (1884). Sur les questions d'ordre: répétitions. Bull. Soc. Fr. Minéral., 7, 89-110; Curie P. (1894). Sur la symétrie dans les phénomènes physiques, symétrie d’un champ électrique et d’un champ magnétique. J. Phys. (Paris), 3, 393-415.).

See also

Curie laws
spherical system
Section 10.1.4 of International Tables of Crystallography, Volume A
Section 1.1.4 of International Tables of Crystallography, Volume D

---