Difference between revisions of "Modulated crystal structure"
From Online Dictionary of Crystallography
TedJanssen (talk | contribs) |
BrianMcMahon (talk | contribs) (Added German and Spanish translations (U. Mueller)) |
||
| (7 intermediate revisions by 3 users not shown) | |||
| Line 1: | Line 1: | ||
| − | + | <font color="blue">Structure cristalline modulée</font> (''Fr''). <font color="red">Modulierte Kristallstruktur</font> (''Ge''). <font color="black">Struttura cristallina modulata</font> (''It''). <font color="purple">変調構造</font> (''Ja''). <font color="green">Estructura cristalina modulada</font> (''Sp''). | |
| − | + | == Definition == | |
| − | |||
| − | |||
| − | |||
| − | |||
A ''modulated crystal structure'' is a density (or atom arrangement) that may be obtained from a density | A ''modulated crystal structure'' is a density (or atom arrangement) that may be obtained from a density | ||
(or atom arrangement) with space-group symmetry by a finite density change (or finite displacement | (or atom arrangement) with space-group symmetry by a finite density change (or finite displacement | ||
| − | of each atom, respectively) that is (quasi)periodic. A function or a displacement field is periodic | + | of each atom, respectively) that is [[Quasiperiodicity|(quasi)periodic]]. A function or a displacement field is periodic |
| − | if it is invariant under a lattice of translations. Then its Fourier transform consists of | + | if it is invariant under a [[lattice]] of translations. Then its Fourier transform consists of |
δ-peaks on a reciprocal lattice that spans the space and is nowhere dense. A quasiperiodic | δ-peaks on a reciprocal lattice that spans the space and is nowhere dense. A quasiperiodic | ||
function has a Fourier transform consisting of δ-peaks on a vector module of finite rank. This means that the peaks may be indexed with integers using a finite number of basis vectors. If the modulation consists of deviations from the basic structure in the positions, | function has a Fourier transform consisting of δ-peaks on a vector module of finite rank. This means that the peaks may be indexed with integers using a finite number of basis vectors. If the modulation consists of deviations from the basic structure in the positions, | ||
| − | the modulation is | + | the modulation is [[displacive modulation|''displacive'']] (see figure). When the probability distribution deviates from |
| − | that in the basic structure the modulation is occupational. | + | that in the basic structure the modulation is ''occupational''. |
| + | |||
| + | [[Image:BO01F05.gif]] | ||
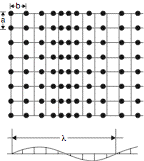
| − | + | Model for a displacively modulated crystal structure. The basic structure is two-dimensional rectangular, with lattice constants ''a'' and ''b'', the modulation wave vector is in the ''b''-direction, the wavelength of the periodic modulation is λ such that λ/''b'' is an irrational number. | |
| − | [[ | + | == See also == |
| + | *[[Displacive modulation]] | ||
| + | *[[Modular crystal structure]] | ||
| − | + | [[Category:Fundamental crystallography]] | |
| − | |||
| − | |||
| − | |||
Latest revision as of 12:58, 16 November 2017
Structure cristalline modulée (Fr). Modulierte Kristallstruktur (Ge). Struttura cristallina modulata (It). 変調構造 (Ja). Estructura cristalina modulada (Sp).
Definition
A modulated crystal structure is a density (or atom arrangement) that may be obtained from a density (or atom arrangement) with space-group symmetry by a finite density change (or finite displacement of each atom, respectively) that is (quasi)periodic. A function or a displacement field is periodic if it is invariant under a lattice of translations. Then its Fourier transform consists of δ-peaks on a reciprocal lattice that spans the space and is nowhere dense. A quasiperiodic function has a Fourier transform consisting of δ-peaks on a vector module of finite rank. This means that the peaks may be indexed with integers using a finite number of basis vectors. If the modulation consists of deviations from the basic structure in the positions, the modulation is displacive (see figure). When the probability distribution deviates from that in the basic structure the modulation is occupational.
Model for a displacively modulated crystal structure. The basic structure is two-dimensional rectangular, with lattice constants a and b, the modulation wave vector is in the b-direction, the wavelength of the periodic modulation is λ such that λ/b is an irrational number.