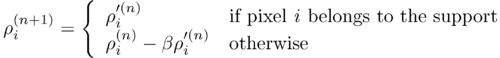Difference between revisions of "Hybrid input-output algorithm"
From Online Dictionary of Crystallography
(+ cat) |
BrianMcMahon (talk | contribs) m (Style edits to align with printed edition) |
||
| Line 3: | Line 3: | ||
An algorithm proposed by R. Fienup in 1982. The original application of the algorithm was the phase retrieval of diffraction patterns of non-periodic objects. However, the algorithm or its components have been used several times also in crystallography. | An algorithm proposed by R. Fienup in 1982. The original application of the algorithm was the phase retrieval of diffraction patterns of non-periodic objects. However, the algorithm or its components have been used several times also in crystallography. | ||
| − | Let <math>\rho^{(n)}</math> be a trial scattering density in the | + | Let <math>\rho^{(n)}</math> be a trial scattering density in the ''n''th iteration cycle. Let <math>\rho'^{(n)}</math> be a density obtained from <math>\rho^{(n)}</math> by Fourier-transforming <math>\rho^{(n)}</math>, replacing all Fourier amplitudes by the experimentally observed amplitudes, and applying inverse Fourier transform. Then the density <math>\rho^{(n+1)}</math> is defined pixel-wise by the following scheme: |
<br>[[Image:CF_4.png|500px|center]]<br> | <br>[[Image:CF_4.png|500px|center]]<br> | ||
Latest revision as of 14:00, 15 May 2017
Definition
An algorithm proposed by R. Fienup in 1982. The original application of the algorithm was the phase retrieval of diffraction patterns of non-periodic objects. However, the algorithm or its components have been used several times also in crystallography.
Let [math]\rho^{(n)}[/math] be a trial scattering density in the nth iteration cycle. Let [math]\rho'^{(n)}[/math] be a density obtained from [math]\rho^{(n)}[/math] by Fourier-transforming [math]\rho^{(n)}[/math], replacing all Fourier amplitudes by the experimentally observed amplitudes, and applying inverse Fourier transform. Then the density [math]\rho^{(n+1)}[/math] is defined pixel-wise by the following scheme:
In crystallography, the support is usually not known [math]\textit{a~priori}[/math], and a dynamical support must be used, [math]\textit{i.e.}[/math] the support is newly defined in each iteration cycle based on a predefined criterion.