Difference between revisions of "Brillouin zone"
From Online Dictionary of Crystallography
AndreAuthier (talk | contribs) |
|||
| Line 1: | Line 1: | ||
| − | <Font color=blue> Zones de Brillouin </Font>(''Fr''). <Font color=red> Brillouin Zonen </Font>(''Ge''). <Font color=green> Zonas de Brillouin </Font>(''Sp''). | + | <Font color=blue> Zones de Brillouin </Font>(''Fr''). <Font color=red> Brillouin Zonen </Font>(''Ge''). <Font color=green> Zonas de Brillouin </Font>(''Sp'').<Font color="black"> Zone di Brillouin </Font>(''It'') |
| + | |||
== Definition == | == Definition == | ||
Revision as of 15:21, 17 March 2006
Zones de Brillouin (Fr). Brillouin Zonen (Ge). Zonas de Brillouin (Sp). Zone di Brillouin (It)
Definition
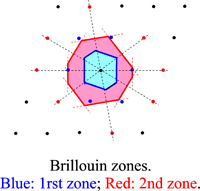
Brillouin zones are used in band theory to represent in reciprocal space the solutions of the wave equations for the propagation of phonons or electrons in solids. The first Brillouin zone is the Wigner-Seitz cell of the reciprocal lattice. It is a polyhedron obtained by connecting a lattice point to its first neighbours and drawing the planes perpendicular to these connecting lines and passing through their midpoints. The second Brillouin zone is obtained by a similar construction but the second-nearest neighbours.
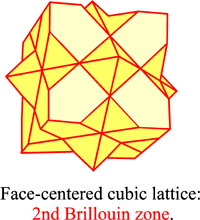
The first Brillouin zone of a face-centered cubic lattice is an cubooctahedron (see Wigner-Seitz cell) and the second Brillouin zone is represented below.
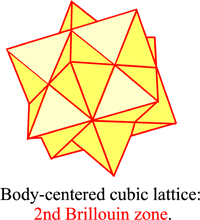
The first Brillouin zone of a body-centered cubic lattice is an rhomb-dodecahedron (see Wigner-Seitz cell) and the second Brillouin zone is represented below.