Difference between revisions of "Bragg's law"
From Online Dictionary of Crystallography
AndreAuthier (talk | contribs) |
AndreAuthier (talk | contribs) |
||
| Line 3: | Line 3: | ||
== Definition == | == Definition == | ||
| + | [[Image:BraggLaw-1.gif|right]] | ||
Bragg's law provides the condition for a plane wave to be diffracted by a family of lattice planes: | Bragg's law provides the condition for a plane wave to be diffracted by a family of lattice planes: | ||
| Line 19: | Line 20: | ||
* '''Reflection from the second plane''' | * '''Reflection from the second plane''' | ||
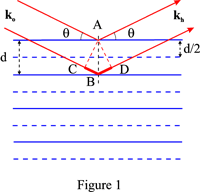
| − | Since the phase of the reflected waves is independent of the position of the pôint scatterer in the plane, the phase difference between the waves reflected by two successive lattice planes is obtained by choosing arbitrarily a scattering point, ''A'', on the first plane and a scattering point, ''b'' on the second plane such that ''AB'' is normal to the planes. If ''C'' and ''d'' are the projections of ''A'' on the incident and reflected wave vectors passing through ''B'', it is clear from | + | Since the phase of the reflected waves is independent of the position of the pôint scatterer in the plane, the phase difference between the waves reflected by two successive lattice planes is obtained by choosing arbitrarily a scattering point, ''A'', on the first plane and a scattering point, ''b'' on the second plane such that ''AB'' is normal to the planes. If ''C'' and ''d'' are the projections of ''A'' on the incident and reflected wave vectors passing through ''B'', it is clear from figure 1 that the path difference between the waves reflected at ''A'' and ''B'', respectively, is: |
<center> | <center> | ||
| Line 45: | Line 46: | ||
</center> | </center> | ||
| − | This fictitious family is associated to the reciprocal lattice vector '''OH''' where ''OH'' = ''n/''d'' = 1/<math>d_{hkl}</math>. The indices of the reflection are: ''hkl''. For instance, the dashed blue lines in Figure 1 correspond to the fistitious planes associated to the second order, ''n'' = 2. | + | This fictitious family is associated to the reciprocal lattice vector '''OH''' where ''OH'' = ''n''/''d'' = 1/<math>d_{hkl}</math>. The indices of the reflection are: ''hkl''. For instance, the dashed blue lines in Figure 1 correspond to the fistitious planes associated to the second order, ''n'' = 2. |
== History == | == History == | ||
Revision as of 05:41, 28 March 2006
Loi de Bragg (Fr). Bragg Gesetz (Ge). Ley de Bragg (Sp). Legge di Bragg (It)
Contents
[hide]Definition
Bragg's law provides the condition for a plane wave to be diffracted by a family of lattice planes:
2 d sin θ = n λ.
where d is the lattice spacing, θ the angle between the wavevector of the incident plane wave, ko, and the lattice planes, λ its wave length and n is an integer, the order of the reflection. It is equivalent to the diffraction condition in reciprocal space and to the Laue equations.
Direct derivation of Bragg's law
- Reflection from the first plane
The scattered waves will be in phase whatever the distribution of the point scatterers in the first plane if the angle of the reflected wave vector, kh, is also equal to θ. This is Snell-Descartes' law of reflection.
- Reflection from the second plane
Since the phase of the reflected waves is independent of the position of the pôint scatterer in the plane, the phase difference between the waves reflected by two successive lattice planes is obtained by choosing arbitrarily a scattering point, A, on the first plane and a scattering point, b on the second plane such that AB is normal to the planes. If C and d are the projections of A on the incident and reflected wave vectors passing through B, it is clear from figure 1 that the path difference between the waves reflected at A and B, respectively, is:
CB + BD = 2 d sin θ
and that the two waves will be in phase if this path difference is equal to n λ where n' is an integer.
- Reflection from the third, etc., planes
If Bragg's relation is satisfied for the first two planes, the waves reflected with wave vector kh will be in phase fo all the planes of the family.
Order of the reflection
Bragg's law may also be written:
2 (d/n) sin θ = λ.
On may then say that a Bragg reflection of order n on a family of lattice planes or order n is equivalent to reflection of order 1 on a family of fictitious, or imaginary, planes of lattice spacing:
[math]d_{hkl}[/math] = d/n
This fictitious family is associated to the reciprocal lattice vector OH where OH = n/d = 1/[math]d_{hkl}[/math]. The indices of the reflection are: hkl. For instance, the dashed blue lines in Figure 1 correspond to the fistitious planes associated to the second order, n = 2.
History
Bragg (1890-1971) presented his derivation of the reflection condition at a meeting of the Cambridge Philosophical Society on 11 November 1912. His paper was published in 1913 (Bragg W.L., 1913, The Diffraction of Short Electromagnetic Waves by a Crystal, Proc. Cambridge Phil. Soc., 17, 43-57. For details, see P. P. Ewald, 1962, IUCr, 50 Years of X-ray Diffraction, Section 5, page 64.
See also