Difference between revisions of "Mapping"
From Online Dictionary of Crystallography
m |
m (Lang) |
||
| Line 1: | Line 1: | ||
| + | <Font color="blue">Transformation </Font>(''It''). <Font color="black">Trasformazione </Font>(''It''). <Font color="purple">写像</Font>(''Ja'') | ||
| + | |||
| + | |||
The term '''mapping''' is often used in mathematics as a synonym of ''function''. In crystallography it is particularly used to indicate a ''transformation''. | The term '''mapping''' is often used in mathematics as a synonym of ''function''. In crystallography it is particularly used to indicate a ''transformation''. | ||
Revision as of 05:49, 4 March 2015
Transformation (It). Trasformazione (It). 写像(Ja)
The term mapping is often used in mathematics as a synonym of function. In crystallography it is particularly used to indicate a transformation.
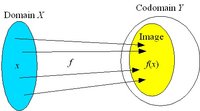
Domain, image and codomain
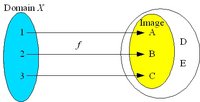
A mapping f of X to Y (f : X → Y) assigns to each element x in the domain X a value y in the codomain Y.The set of values f(X) = { f(x) : x in X } is the image of the mapping. The image may be the whole codomain or a proper subset of it.
For an element y in the image of f, the set { x in X : f(x) = y } of elements mapped to y is called the preimage of y, denoted by f -1{y}. Also, the single elements in f -1{y} are
called preimages of x.
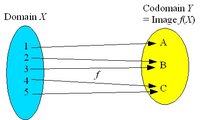
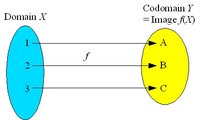
Surjective, injective and bijective mappings
The mapping f is surjective (onto) if the image coincides with the codomain. The mapping may be many-to-one because more than one element of the domain X can be mapped to the same element of the codomain Y, but every element of Y has a preimage in X. A surjective mapping is a surjection.
If the codomain of an injective mapping f is restricted to the image f(X), the resulting mapping is a bijection from X to f(X).