Mapping

The term mapping is often used in mathematics as a synonym of function. In crystallography it is particularly used to indicate a transformation.

Domain, image and codomain

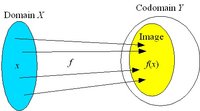
Definition of domain, image and codomain

A mapping f of X to Y (f : X → Y) assigns to each element x in the domain X a value y in the codomain Y.

The set of values f(X) = { f(x) : x in X } is the image of the mapping. The image may be the whole codomain or a proper subset of it.
For an element y in the image of f, the set { x in X : f(x) = y } of elements mapped to y is called the preimage of y, denoted by f ^-1{y}. Also, the single elements in f ^-1{y} are called preimages of x.

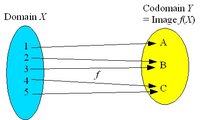
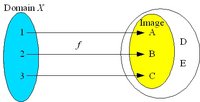
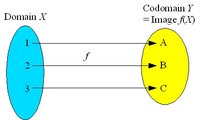
Surjective, injective and bijective mappings

In a surjective mapping the image is the whole codomain

The mapping f is surjective (onto) if the image coincides with the codomain. The mapping may be many-to-one because more than one element of the domain X can be mapped to the same element of the codomain Y, but every element of Y has a preimage in X. A surjective mapping is a surjection.

An injective mapping is a one-to-one mapping

The mapping f is injective (one-to-one mapping) if different elements of the domain X are mapped to different elements in the codomain Y. The image does not have to coincide with the codomain and therefore there may be elements of Y that are not mapped to some elements of X. An injective mapping is an injection.

A bijective mapping is a one-to-one correspondence

The mapping f is bijective (one-to-one correspondence) if and only if it is both injective and surjective. Every element of the codomain Y has exactly one preimage in the domain X. The image coincides with the codomain. A bijective mapping is a bijection.

If the codomain of an injective mapping f is restricted to the image f(X), the resulting mapping is a bijection from X to f(X).