Orientation matrix
From Online Dictionary of Crystallography
Revision as of 14:36, 14 July 2021 by BrianMcMahon (talk | contribs) (Minor typos, tidied references)
In a diffraction experiment, the orientation matrix describes the orientation of the single crystal in space. The matrix relates the reciprocal axes of the crystal with the Cartesian coordinate system of the diffractometer. In order to calculate the impact position of a reflection hkl on the detector, one needs the orientation matrix and the setting angles of the goniostat motors. In most cases, the determination of the orientation matrix is the first step in a diffraction experiment and is based on a rather small number of reflections. In a later stage, the orientation matrix can be refined on a larger number of reflections.
Other commonly used names for the orientation matrix are reciprocal axes matrix and UB matrix.
The orientation matrix is a product of the matrix U and the matrix B. The matrix B transforms a reciprocal lattice vector into an orthonormal coordinate system. The matrix B has the following property: B^T B = G^{-1} (G^{-1} is the reciprocal space metric tensor). The matrix U is a rotation matrix and relates the orthonormal coordinate system of the crystal to the orthonormal coordinate system of the diffractometer.
Matrix B can be created in an infinite number of ways, and different computer programs use different definitions for the diffractometer coordinate system. Care needs to be taken how the orientation matrix is defined in each individual case.
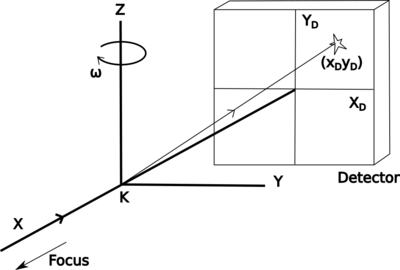
Example coordinate system
Definition of the diffractometer coordinate system in the EVAL software (Duisenberg et al., 2003).
Example orientation matrix
In this example definition, the reciprocal axes matrix is given as
R = \left( \begin{array}{lll} a^\star_x & b^\star_x & c^\star_x \\ a^\star_y & b^\star_y & c^\star_y \\ a^\star_z & b^\star_z & c^\star_z \end{array} \right)
It is thus straightforward to extract the reciprocal axes from this matrix. a^\star corresponds to the first column, b^\star to the second, c^\star to the third. The subscripts x, y, and z indicate the Cartesian coordinates of the diffractometer.
References
- Busing, W. R. and Levy, H. A. (1967). Acta Cryst. 22, 457-464.
- Duisenberg, A. J. M., Kroon-Batenburg, L. M. J. and Schreurs, A. M. M. (2003). J. Appl. Cryst. 36, 220-229. An intensity evaluation method: EVAL-14.
- Luger, P. (1980). Modern X-ray Analysis on Single Crystals, Berlin, New York: de Gruyter, pp. 176-183.
- E. Prince (1994). Mathematical Techniques in Crystallography and Materials Science (2nd ed.), Berlin: Springer, pp. 54-56.