Miller indices

Indices de Miller (Fr.). Indices de Miller (Sp.)

Definition

Direct space

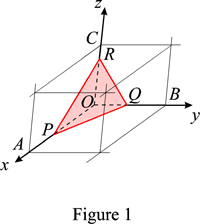
Planes of a given family of lattice planes with Miller indices h, k, l make intercepts OP = C a/h, OQ = C b/k, and OR = C c/l with the unit-cell axes OA = a, OB = b, and OC = c (see Figure 1), where h, k, l are prime integers and C is a constant integer; the planes of the family are denoted (hkl). This property results from the law of rational indices. The Miller indices of the equivalent faces of a crystal form are denoted by {hkl}. The variation of the orientation of the planes with the ratios of the Miller indices is illustrated in the attached examples. The equation of the planes of the family is:

hx + ky + lz = C

Reciprocal space

The reciprocal lattice vector associated to the family of lattice planes is OH = h a* + k b* + l c*, where a*, b*, c* are the reciprocal lattice basis vectors. OH is perpendicular to the family of lattice planes and OH = 1/d where d is the lattice spacing of the family.

Bravais-Miller indices (hexagonal axes)

In the case of an hexagonal lattice, one uses four axes, a₁, a₂, a₃, c and four indices, (hkil), called Bravais-Miller indices, where h, k, i, l are again inversely proportional to the intercepts of a plane of the family with the four axes. The indices h, k, i are cyclically permutable and are related by

h + k + i = 0

Behaviour in a change of basis

In a change of basis the Miller indices h, k, l transform like the basis vectors a, b, c and are for that reason covariant quantities.

Rhombohedral crystals

The Miller indices h_R, k_R, l_R referred to rhombohedral axes are related to the corresponding indices, h_H, k_H, i_H,l_H reffered to hexagonal axes by:

hH = kR - lR	;	hR = ⅓(- kH + iH + lH)
kH = lR - hR	;	kR = ⅓(hH - iH + lH)
iH = hR - kR	;	lR = ⅓(- hH + kH + lH)
lH = hR + kR + lR

History

The Miller indices were first introduced, among others, by W. Whevell in 1829 and developed by W.H. Miller, his successor at the Chair of Mineralogy at Cambridge University, in his book A treatise on Crystallogtaphy (1839) - see Historical Atlas of Crystallography (1990), edited by J. Lima de Faria, published for the International Union of Crystallography by Kluwer Academic Publishers, Dordrecht.

See also

law of rational indices
reciprocal lattice

---