Dynamical theory
From Online Dictionary of Crystallography
Revision as of 14:13, 15 April 2024 by BrianMcMahon (talk | contribs)
Théorie dynamique (Fr). Dynamische Theorie (Ge). Teoria dinamica (It). 動力学的回折理論 (Ja). Teoria dynámica (Sp).
Overview
In the geometrical, or kinematical, theory, the amplitudes diffracted by a three-dimensional periodic assembly of atoms (Laue) or by a stack of planes (Darwin) is derived by adding the amplitudes of the waves diffracted by each atom or by each plane, simply taking into account the optical path differences between them, but neglecting the interaction of the propagating waves and matter. This approximation is not compatible with the law of conservation of energy and is only valid for very small or highly imperfect crystals. The purpose of the dynamical theory is to take this interaction into account. There are three forms of the dynamical theory:
Darwin's theory
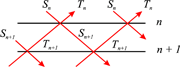
Charles Darwin (the grandson of the author of the theory of evolution) takes into account the interaction between the waves partially transmitted ([math] S_n, \ S_{n+1} [/math]) and partially reflected ([math] T_n, \ T_{n+1} [/math]) at the successive atomic planes, n, n+1 etc. by recurrence equations.
Ewald's theory
Paul Ewald solves the propagation equation deduced from Maxwell's equations in a medium constituted of a three-dimensional periodic array of discrete scattering dipoles.
Laue's theory
Max von Laue considers that the negative and positive electric charges are distributed in a continuous way throughout the volume of the crystal. Since the crystal must be neutral, they cancel out and the local electric charge and density of current are equal to zero. The interaction of electromagnetic waves with the positive charges is neglected as a first approximation in the usual dynamical theory, although resonant nuclear scattering of X-rays exists and has been observed for γ- and X-rays. The medium is polarized under the influence of the electric field and E = D/ε, where D is the electric displacement and [math]\epsilon = \epsilon_0(1 + \chi) [/math] varies with the space coordinates. The continuous dielectric susceptibility, or polarizability, χ, takes into account the interaction of the electromagnetic radiation with the distribution of electric charges. By eliminating D, the magnetic field, H, and the magnetic induction, B, in Maxwell's equations, one obtains a wave equation for E:
curl curl [math]\mathbf{E} - 4 \pi ^2 k^2 [1 + \chi(\mathbf{r})] \mathbf{E} = 0 [/math]
where k = 1/λ is the wave number in vacuum.
While div E is equal to zero, this is not true for div D, and the electric displacement is in general a more suitable quantity than the electric field because it simplifies the description of the polarization states of the field inside the crystal. It is used in Laue's formulation of the dynamical theory. With a small approximation the propagation equation becomes:
[math]\Delta \mathbf{D}[/math] + curl curl [math]\chi (\mathbf{r})\mathbf{E} + 4 \pi ^2 k^2 \mathbf{D} = 0.[/math]
For material waves such as electrons or neutrons, the propagation equation is derived from Schrödinger's equation:
[math] \Delta \Psi + 4\ \pi^ 2k^2\left[1 + \chi (\mathbf{r})\right]\Psi = 0 [/math]
where
- [math]\chi(\mathbf{r}) = \varphi (\mathbf{r})/ W [/math] in the case of electron diffraction [[math]\varphi (\mathbf{r})[/math], potential in the crystal and W accelerating voltage],
- [math]\chi(\mathbf{r}) = - 2mV(\mathbf{r})/h^2k^2 [/math] in the case of neutron diffraction [[math]V(\mathbf{r}[/math]), Fermi pseudo-potential].
The purpose of the dynamical theory is to solve the propagation equation taking into account the boundary conditions. It offers many similarities with the band theory of solids. The difference is that, in the band theory, one studies the possible energies of electrons as a function of their wavenumber while, in diffraction theory, the energy is constant and one looks for the possible positions of the wave vectors in reciprocal space.
History
- Laue's geometrical theory: Friedrich, W., Knipping, P. and Laue, M. von (1912). Sitzungsberichte der Kgl. Bayer. Akad. der Wiss., 303-322, reprinted in Ann. Phys. (1913), 41, 971. Interferenz-Erscheinungen bei Röntgenstrahlen.
- Darwin's geometrical theory: Darwin, C. G. (1914). Philos. Mag., 27, 315-333. The Theory of X-ray Reflection.
- Darwin's geometrical theory: Darwin, C. G. (1914). Philos. Mag., 27, 675-690. The Theory of X-ray Reflection. Part II.
- Ewald's dynamical theory: Ewald, P. P. (1917). Ann. Physik, 54, 519-597, Zur Begründung der Kristalloptik. III. Die Kristalloptik der Röntgenstrahlen.
- Laue's dynamical theory: Laue, M. von (1931). Ergeb. Exakt. Naturwiss. 10, 133-158, Die dynamische Theorie der Röntgenstrahlinterferenzen in neuer Form and (1931). Röntgenstrahl-Interferenzen. Akademische Verlagsgesellschaft, Frankfurt am Main.
For a detailed eaccount of the historical developments, see P. P. Ewald (1962), 50 Years of X-ray Diffraction, Utrecht: IUCr/Oosthoek, Section 5, p. 64, and A. Authier (2013), The Early Days of X-ray Diffraction, Oxford: IUCr/Oxford University Press, Chapters 6 and 9.
See also
- Chapter 5.1 of International Tables for Crystallography, Volume B for X-rays
- Chapter 5.2 of International Tables for Crystallography, Volume B for electrons
- Chapter 5.3 of International Tables for Crystallography, Volume B for neutrons
- Authier, A. (2005). Dynamical Theory of X-ray Diffraction. Oxford: IUCr/Oxford University Press