Difference between revisions of "Wigner-Seitz cell"
From Online Dictionary of Crystallography
BrianMcMahon (talk | contribs) m |
m (→See also: ITA 6th edition) |
||
| Line 17: | Line 17: | ||
== See also == | == See also == | ||
| + | *Section 3.1.1.4 of ''International Tables of Crystallography, Volume A'', 6<sup>th</sup> edition | ||
| + | *Section 1.5 of ''International Tables of Crystallography, Volume B'' | ||
| + | *Sections 1.2 and 2.2 of ''International Tables of Crystallography, Volume D'' | ||
| − | + | [[Category:Fundamental crystallography]] | |
| − | |||
| − | |||
| − | |||
| − | [[Category:Fundamental crystallography]] | ||
Revision as of 17:02, 11 April 2017
Maille de Wigner-Seitz (Fr). Wigner-Seitz Zell (Ge). Celda de Wigner-Seitz (Sp). Cella di Wigner-Seitz (It)
Definition
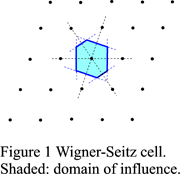
The Wigner-Seitz cell is a a polyhedron obtained by connecting a lattice point P to all other lattice points and drawing the planes perpendicular to these connecting lines and passing through their midpoints (Figure 1). The polyhedron enclosed by these planes is the Wigner-Seitz cell. This construction is called the Dirichlet construction. The cell thus obtained is a primitive cell and it is possible to fill up the whole space by translation of that cell.
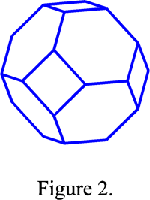
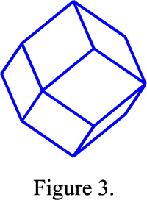
The Wigner-Seitz cell of a body-centred cubic lattice I is a cuboctahedron (Figure 2) and the Wigner-Seitz cell of a face-centred cubic lattice F is a rhomb-dodecahedron (Figure 3). In reciprocal space this cell is the first Brillouin zone. Since the reciprocal lattice of body-centred lattice is a face-centred lattice and reciprocally, the first Brillouin zone of a body-centred cubic lattice is a rhomb-dodecahedron and that of a face-centred cubic lattice is a cuboctahedron.
The inside of the Wigner-Seitz cell has been called domain of influence by Delaunay (1933). It is also called Dirichlet domain or Voronoi domain. The domain of influence of lattice point P thus consists of all points Q in space that are closer to this lattice point than to any other lattice point or at most equidistant to it (such that OP ≤ |t - OP| for any vector t ∈ L).
See also
- Section 3.1.1.4 of International Tables of Crystallography, Volume A, 6th edition
- Section 1.5 of International Tables of Crystallography, Volume B
- Sections 1.2 and 2.2 of International Tables of Crystallography, Volume D